Eva and Mario - Ticket Sales

What can you do with this video?

Download the video here.

Eva and Mario - An Adventure in Rock Collecting

What could you do with this video?

Download the video here.

Linear Equation Posters

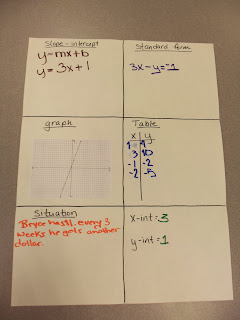
 As you know, I recently attended a co-teaching conference.  Once of the things that I learned from the conference was to get the students up and moving if possible.  That's how this poster activity was born.

I created 6 posters where difference pieces of information were given on each one.  The black ink is what I wrote.  When the students came into the room that day I had the posters is different locations.  I put the students into groups of 2 or 3.  Each group received a different color marker, this way I know which group wrote what.

The students had 2 minutes to pick one of the five empty rectangles and fill it in as it related to what I wrote in black.  

Then the students moved to the next poster and filled in one of the four rectangles that were empty.  

As they moved from poster to poster, they were also instructed to look at the work of previous groups and make (appropriate) comments or corrections.  Writing "you're an idiot" is not appropriate.

Given: x- and y-intercept

Given: Slope-Intercept Form

Given: Situation

Given: Standard Form

Given: a table of values

Given:  the graph of a line

Reflection:

Pros:  The students were up and moving, I could see what they were writing,  I could easily see and hear misunderstandings, and the students were having good discussions about algebra.

Cons:  I put the posters too close together because my wall space is limited.  Next time I will make use of my table.  Just because they're posters doesn't mean they have to hang on the wall.  I had the posters about 4 feet apart, but this meant the entire class was crowed in the front of my room.

One misunderstanding that showed its ugly head -->  Students didn't know the different between the x-intercept and identifying what x represents (such as the situation).

Barbie Bungee Jumping

I've been toying around with this activity for a few years now, but I've never had a class that I trusted enough to cooperate and do this.  This year the students are no different, however, I am co-teaching this class and there are two more eyes to help with crowd control.  

This activity was used with a 9th-grade Algebra 1 class.

The activity in a nut shell:

1) Students determine how far Barbie bungees with up to 10 rubber bands.

2) Students determine how many rubber bands are needed to have her jump from a higher height, but using their knowledge of regression equations, slope, and y-intercept.

3) Finally, students bungee their Barbies from that height.

The original idea came from Illumination from NCTM.

Here is the packet that I created for my students.

We started in the classroom and the students used up to 10 rubber bands.  A few problems that I noticed along the way.

- Students had difficulty finding average.  Yes, average.  They completely forgot about the order of operations with the calculator and would type this in:  50 + 49 + 51 / 3.  They neglected to use parenthesis or the ENTER button.

- The students assumed that it's okay to round.  No! No! No!  Not when Barbie's life depends on it.

 - The students did not double check each other's work.  One student solve the equation incorrectly and the rest of the group assumed she did it right.  

The big day - when it finally stopped raining we were able to bungee the dolls from a 14-foot height.

The results - Two of the groups did well. Their Barbie came within inches of the ground.  Three of the groups didn't have enough rubber bands for the reasons listed above.  Three of the other groups "killed" their Barbies with too many rubber bands.  I believe their mistake was in the classroom and not accurately measuring the bungee distance.

Reflections:

I will do this activity again.  Not only did it help students with concepts of linear equation, but it was a nice review of estimation, average, and rounding.

The students were more well-behaved that I thought they would be.  I believe this is because they were very interested in the activity.  This helps with one of my problems - being afraid of my students.  Not afraid for myself, but afraid of lack of cooperation.  Perhaps if I would show some trust more often, I would see more cooperation.  Maybe.

What I would do differently next time.
I will need to emphasize the importance of not rounding and the importance of measuring accurately.

Student Journals

Classroom Products Warehouse just sent me an e-mail that offered my 10 free math journals with any purchase, and no minimum requirement.  Here is a link to check out these math journals.  If you haven't realized by now, I like to create my own classroom products for next to nothing.  So, I checked out the journals they were offering and decided to see if I could duplicate them.  Here is the result:

I intentionally leave the front cover blank so that students can create their own design.  I do insist that their name is somewhere on the front.

Inside I have lines for journaling and a grid for graphing.  I also like to leave out the axes.  I feel that students should decide if all four quadrants are necessary or if they should only use the first.


So, here's how you make these things.  First print out the journals.  The first 2 pages should be back-to-back, as well as the last two pages.

I printed page numbers at the bottom of each page.  Take the paper with pages 4, 7, 8, and 3 on it and cut it like the photo above.  Make sure to make cuts at the top and bottom.

Take the other paper (pages 2, 5, 6, and 1) and cut a line down the middle like the photo above.

Now, let's go back to the first paper (pages 4, 7, 8, and 3) and hold page 4 like this (4 is inside the roll, 3 is on the outside).

Take the first paper (the one in your hand) and insert it into the second paper.  The second paper should have pages 2 and 5 facing up.

Pull that paper all the way through and there you have it.  Your very own math journal on the cheap.

Tire Calibration

I posed a question to my "lower" pre-calc class today.  I started by telling them that the problem was really hard and that I wanted to determine who the "smarty-pants" in the class were.  This is a class full of students who won't quite make it to Calculus.  Most of them have trouble understanding material, they prefer to memorize.  So I take special care of this class and make sure I show them why.  I rarely teach them any tricks as they will rely of those and forget why.  Anyway, below is the problem and I am proud to say that about half of the students got a correct solution and beyond that they were proud of themselves too.

As you are driving down a road in your car you see that your speedometer shows a velocity of 45 mph.  However, you know that you have the wrong tires on your car.  The tires you have on have a radius of 18 inches and your tires should have a radius of 16 inches.  This means that even though your speedometer reads 45 mph, you are truly going faster.  What is the true linear speed of your car?  Show and explain all of your work.

Hot Dog Cookers

Last school year, while looking over my curriculum map, I came across my unit on conic sections.  The first question that crossed my mind was, "Why is this important to learn?"  In my entire life, I have never used conic sections unless I was teaching it.  It then became my mission to find a reason for my students to learn conic sections.

In my research I found parabolic solar cookers and I was hooked.  I ran the idea past my colleague, who teaches the same course and we immediately went to work.  During the last in-service day of the school year we constructed this solar cooker.  In the afternoon we purchased a pack of hotdogs and set out to see if this was an idea for our students.

After eating our HOT hot dog, we started to devise a plan in our heads, and "Hot Dog Day" was born. This May our pre-calc classes will be taking their cookers outside to cook, you guessed it, hot dogs.  

Have I done this yet?  No, but I was too excited to not tell you about it.  I will post again later but wanted to throw this out there now.
Currently our students are determining their focus point and just how exactly they are going to construct their cooker.  Our students are excited beyond belief and, for the first year in the history of teaching this class, they understand the importance of conic sections.  I too have found a reason to learn about them.
Now, I need a reason to make ellipses important....hmmm...